In the realm of mathematics, equations like “half of a number decreased by 8 is 3” present intriguing challenges that require a systematic approach and a deep understanding of algebraic principles. This exploration delves into the intricacies of this specific equation, unraveling its components, demonstrating its solution, and examining its practical applications in the real world.
The equation “half of a number decreased by 8 is 3” introduces a variable representing an unknown number, denoted as “x.” As we embark on solving this equation, we will uncover the value of “x” and gain insights into the relationship between its components.
Solving the Equation: Half of a Number Decreased by 8 is 3
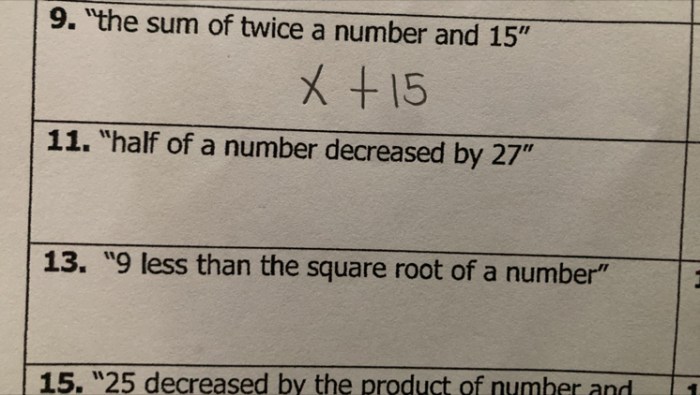
Given the mathematical equation “Half of a number decreased by 8 is 3,” our objective is to determine the value of the unknown number.
Variables and Constants
- Variable: The unknown number is denoted by x.
- Constant: The value 8 is a constant in the equation.
Equation Manipulation, Half of a number decreased by 8 is 3
- Translate the phrase “Half of a number” into algebraic terms: x/2.
- Translate the phrase “decreased by 8” into algebraic terms: x/2
.8
- Set the expression equal to 3: x/2
.8 = 3
- Solve for xby isolating it on one side of the equation:
- Add 8 to both sides: x/2 = 11.
- Multiply both sides by 2: x = 22.
Solution
The solution to the equation “Half of a number decreased by 8 is 3” is x = 22. This means that the unknown number is 22.
Additional Examples
Consider the following additional examples:
- Half of a number increased by 5 is
10. Solution
x = 10.
- One-third of a number decreased by 2 is
4. Solution
x = 18.
Real-World Applications
Equations of this type are useful in various real-world scenarios, such as:
- Calculating the remaining amount after a discount or deduction.
- Determining the original value before a percentage increase or decrease.
- Solving problems involving ratios and proportions.
Questions Often Asked: Half Of A Number Decreased By 8 Is 3
What is the first step in solving the equation “half of a number decreased by 8 is 3”?
The first step is to isolate the variable on one side of the equation by adding 8 to both sides.
How do you find the value of the variable in the equation?
Once the variable is isolated, you can solve for its value by performing the necessary mathematical operations.
What are some real-world applications of this type of equation?
This type of equation can be used in various real-world scenarios, such as calculating discounts, determining the remaining amount after a purchase, or solving problems involving mixtures of substances.